.ipynb .pdf

repository open issue suggest edit

Binder Colab Live Code

Contents

Derivations for moments of univariate normal distribution

Standard Normal

Expectation

$$\begin{array}{r}\begin{array}{c}X\sim p(x)\\ \sim \mathcal{N}(0,1)\\ =\frac{1}{\sqrt{2\pi }}{e}^{(-1/2)x^2}\\ E_X[x]={\int }_{-\mathrm{\infty }}^{\mathrm{\infty }}\frac{1}{\sqrt{2\pi }}{e}^{(-1/2)x^2}\cdot xdx\end{array}\end{array}$$

Solve indefinite integral, $$\begin{array}{l}I=\int \frac{1}{\sqrt{2\pi }}{e}^{-x^2/2}\cdot xdx\\ y=\frac{-x^2}{2}\Rightarrow dy=-xdx\\ \therefore I=\int \frac{-1}{\sqrt{2\pi }}{e}^{y}dy=\frac{-1}{\sqrt{2\pi }}\int e^{y}dy=\frac{-1}{\sqrt{2\pi }}{e}^y\end{array}$$Substituteback$$I=\frac{-1}{\sqrt{2\pi }}{e}^{-x^2/2}$$Definiteintegralis$\left.\frac{-1}{\sqrt{2 \pi}} e^{-x^{2}}\right|_{-\infty} ^{\infty}=\frac{-1}{\sqrt{2 \pi}}\left(e^{-\infty}-e^{-\infty}\right)=0$

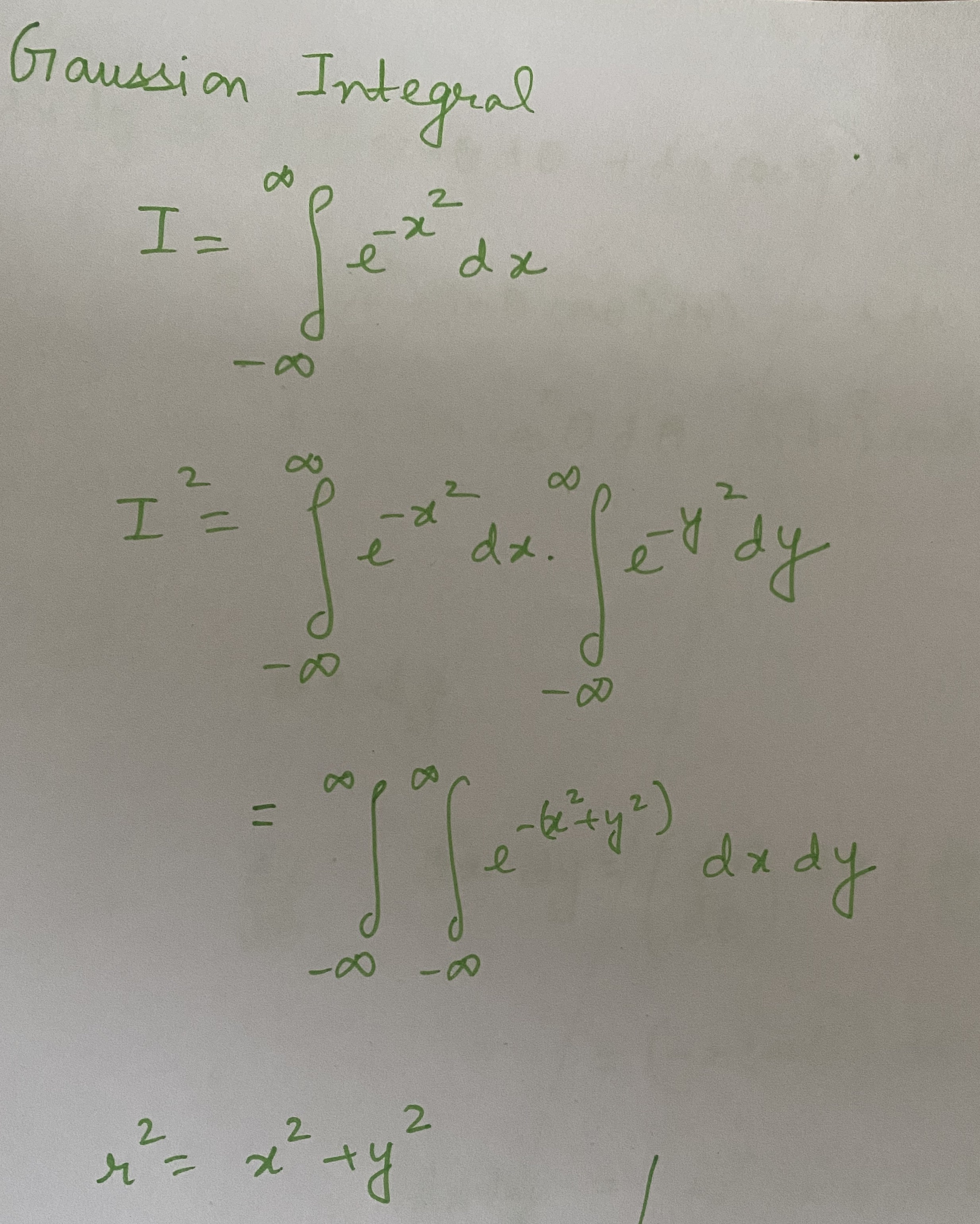
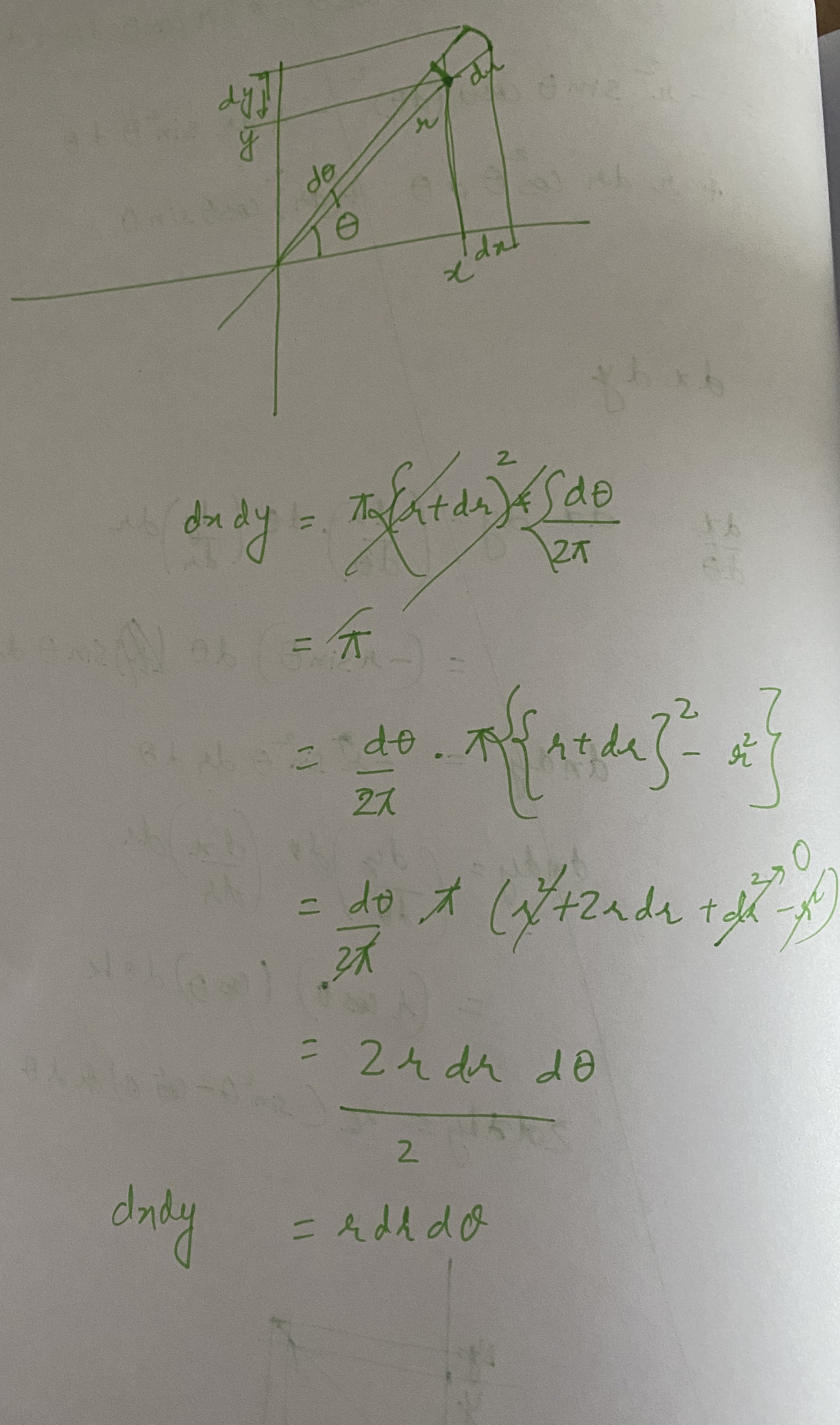
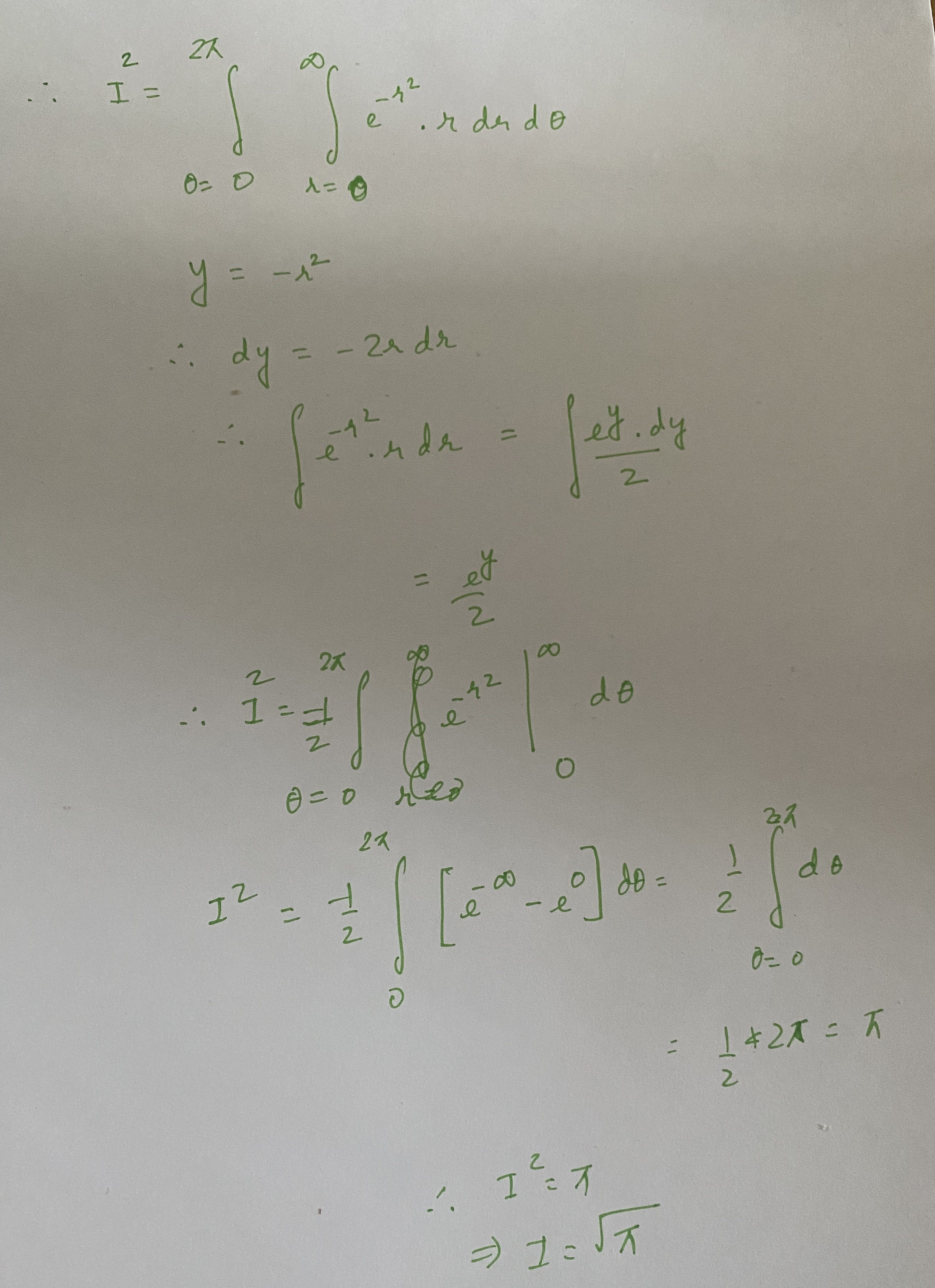
Variance

Affine Transformation of Standard Normal

References

1. https://www.youtube.com/watch?v=U-13q74uvTo

2. https://www.youtube.com/watch?v=mrcRODAx-Vk

3. https://en.wikipedia.org/wiki/Gaussian_integral

4. https://www.youtube.com/watch?v=zbHRUnR9F-4 (Very detailed!)

5. https://www.youtube.com/watch?v=l27xKSNad2Y (Very intuitive double integral explanation)

previous

Properties of RV

next

Multivariate Normal Distribution: Introduction